The factors of 20 are the numbers 1, 2, 4, 5, 10, 20, -1, -2, -4, -5, -10, and -20. As you can see, the total number of factors 20 has is 12. Make the following observations!
- The numbers 1, 2, 4, 5, 10, 20 are called positive factors of twenty
- The numbers -1, -2, -4, -5, -10, and -20 are called negative factors of twenty
- Among the positive factors of twenty, 1 is the smallest factor of 20 and 20 is the biggest factor of 20
- Among the negative factors of twenty, -20 is the smallest factor of 20 and -1 is the biggest factor of 20
How to easily find the factors of 20
By definition, the numbers a and b are called factors of ab and notice that the product ab can also be written as a × b.
Since 20 can also be written as 4 × 5, 4 and 5 are factors of 20.
Therefore, to find all the positive factors of 20, just find all pairs of positive integers when multiplied will give 20.
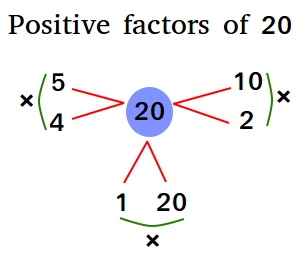
As you can see these pairs are (1,20), (2,10), and (4,5). If a blue circle represents a positive number, you can write the factors as arrays as shown below.
●●●●●●●●●●●●●●●●●●●●
●●●●●●●●●●
●●●●●●●●●●
●●●●
●●●●
●●●●
●●●●
●●●●
To find the negative factors of 20, just find all pairs of negative integers when multiplied will give 20.
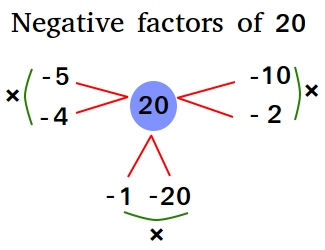
As you can see these pairs are (-1,-20), (-2,-10), and (-4,-5). If a red circle represents a negative number, you can write the factors as arrays as shown below.
●●●●●●●●●●●●●●●●●●●●
●●●●●●●●●●
●●●●●●●●●●
●●●●
●●●●
●●●●
●●●●
●●●●
How to find the factors of 20 by doing division
Just try to find all the numbers smaller than 20 that can divide 20 evenly. A number will divide 20 evenly if the remainder is 0 after you divide 20 by that number. Starting with 1, we show below all the possible division problems that can help us find the positive factors of 20. Just do something similar to find the negative factors of 20.
20/1 = 1 × 20 + 0 (Since the remainder is 0, the factors are 1 and 20)
20/2 = 2 × 10 + 0 (Since the remainder is 0, the factors are 2 and 10)
20/3 = 3 × 6 + 2 (Since the remainder is 2, there are no factors)
20/4 = 4 × 5 + 0 (Since the remainder is 0, the factors are 4 and 5)
20/5 = 5 × 4 + 0 (Since the remainder is 0, the factors are 5 and 4)
20/6 = 6 × 3 + 2 (Since the remainder is 2, there are no factors)
20/7 = 7 × 2 + 6 (Since the remainder is 6, there are no factors)
20/8 = 8 × 2 + 4 (Since the remainder is 4, there are no factors)
20/9 = 9 × 2 + 2 (Since the remainder is 2, there are no factors)
20/10 = 10 × 2 + 0 (Since the remainder is 0, the factors are 10 and 2)
20/11 = 11 × 1 + 9 (Since the remainder is 9, there are no factors)
20/12 = 12 × 1 + 8 (Since the remainder is 8, there are no factors)
20/13 = 13 × 1 + 7 (Since the remainder is 7, there are no factors)
20/14 = 14 × 1 + 6 (Since the remainder is 6, there are no factors)
20/15 = 15 × 1 + 5 (Since the remainder is 5, there are no factors)
20/16 = 16 × 1 + 4 (Since the remainder is 4, there are no factors)
20/17 = 17 × 1 + 3 (Since the remainder is 3, there are no factors)
20/18 = 18 × 1 + 2 (Since the remainder is 2, there are no factors)
20/19 = 19 × 1 + 1 (Since the remainder is 1, there are no factors)
20/20 = 20 × 1 + 0 (Since the remainder is 0, the factors are 20 and 1)
Write down the numbers in blue without repetition to find the factors.
Tips and tricks that can help us quickly find the factors of 20
The number 1 is a factor of every number. Therefore, 1 is a factor of 20 as well.
Every number has at least 2 factors, 1 and the number itself. Therefore, 20 is also a factor of 20.
All even numbers have 2 as one of their factors. Since 20 is even, 2 is also a factor of 20.
All numbers which end in 0 will have 5 and 10 as their factors. Since 20 ends in 0, 5 and 10 are factors.
How to find the prime factors of 20
The prime factors of 20 are all the prime numbers, taken from the factors of 20, that are multiplied to get 20.
A factor tree of 20 can be very useful when looking for the prime factors of 20.
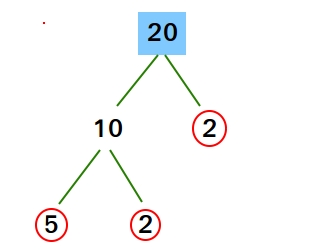
The prime factors are the numbers with the red circle around them. The prime factors are
2, 2, and 5.
Indeed 2 × 2 × 5 = 20
