By Meredith Dadigan Abel, K-5 IM Certified® Facilitator
When teachers come back from summer break, there is a magical feeling in the air. For me, it’s like the feeling that accompanies the first snowfall of the season. Teachers feel refreshed, renewed, and filled with hope for what’s to come. This time of rejuvenation and anticipation is a perfect time for instructional leaders to capitalize on professional learning for teachers.
In-service days just before school starts often dedicate time for teachers to map out the scope and sequence of their curriculum, making sure to include ample time for centers, section checkpoints, and end-of-unit assessments. It is just as important, although often overlooked, to strategically plan how to co-create math communities with their students.
Providing teachers with this time aligns with two of the key pillars that the IM Classroom is built upon: community and leaders. This practice demonstrates that school leaders understand the impact a strong math community can have on teaching and learning all year long.

Author Meredith Abel and fellow teacher Pablo Gomez discuss collected data and adjusting instruction to meet the needs of their math communities.
Reflect on the Past to Plan for the Future
An important first step in this planning process is taking time to reflect on the previous year’s math community and consider its strengths and opportunities for improvement. Teachers can consult IM’s guidance in “Key Structures in this Course,” which highlights various teacher moves that support building a strong math community. They can contemplate which teacher moves they would like to continue or improve upon and which new moves they would like to incorporate.
One way to add additional value to math community planning is to incorporate classroom observations of future students, which requires starting the process at the end of a school year. Unlike an unmarred canvas of freshly fallen snow, students beginning a new school year are not blank slates. They enter the classroom with valuable experiences and knowledge from their previous math communities, bringing who they are and what they know as capable learners of mathematics. At the end of a school year, I always observed the classrooms of my future students. I noticed the elements of their math communities that were flowing and which areas would require extra time and attention when they entered my classroom. While the end of the year can be chaotic, finding coverage for teachers to observe their peers and future students can make a tremendous difference in planning for a strong math community for the following year.
Routines and Procedures
Teachers have a lot to think about when planning to co-create a math community with their students. The most meaningful parts of creating a math community are sometimes the simplest yet most easily forgotten: routines and procedures. Intentional routines and procedures that are consistently implemented from the first day of school will maximize instructional minutes and positively impact the math community all year long.
Because each classroom is unique, the first step is to identify which routines and procedures it needs to run smoothly. For example, teachers and grade-level teams may consider how to transition to and from different partnerships or groups, how to participate in different partnerships, and how to use math tools together.
Once identified, the team can analyze each routine or procedure to consider what knowledge and skills students will need for each task. Creating documents similar to the example below will help teachers plan for and explicitly teach routines and procedures throughout the current school year and in future years. The more these are practiced, the more they become like muscle memory for students. When teachers spend time on routines and procedures, students are enabled to perform them with automaticity, allowing them to focus on having authentic math conversations and fostering an inclusive math community.
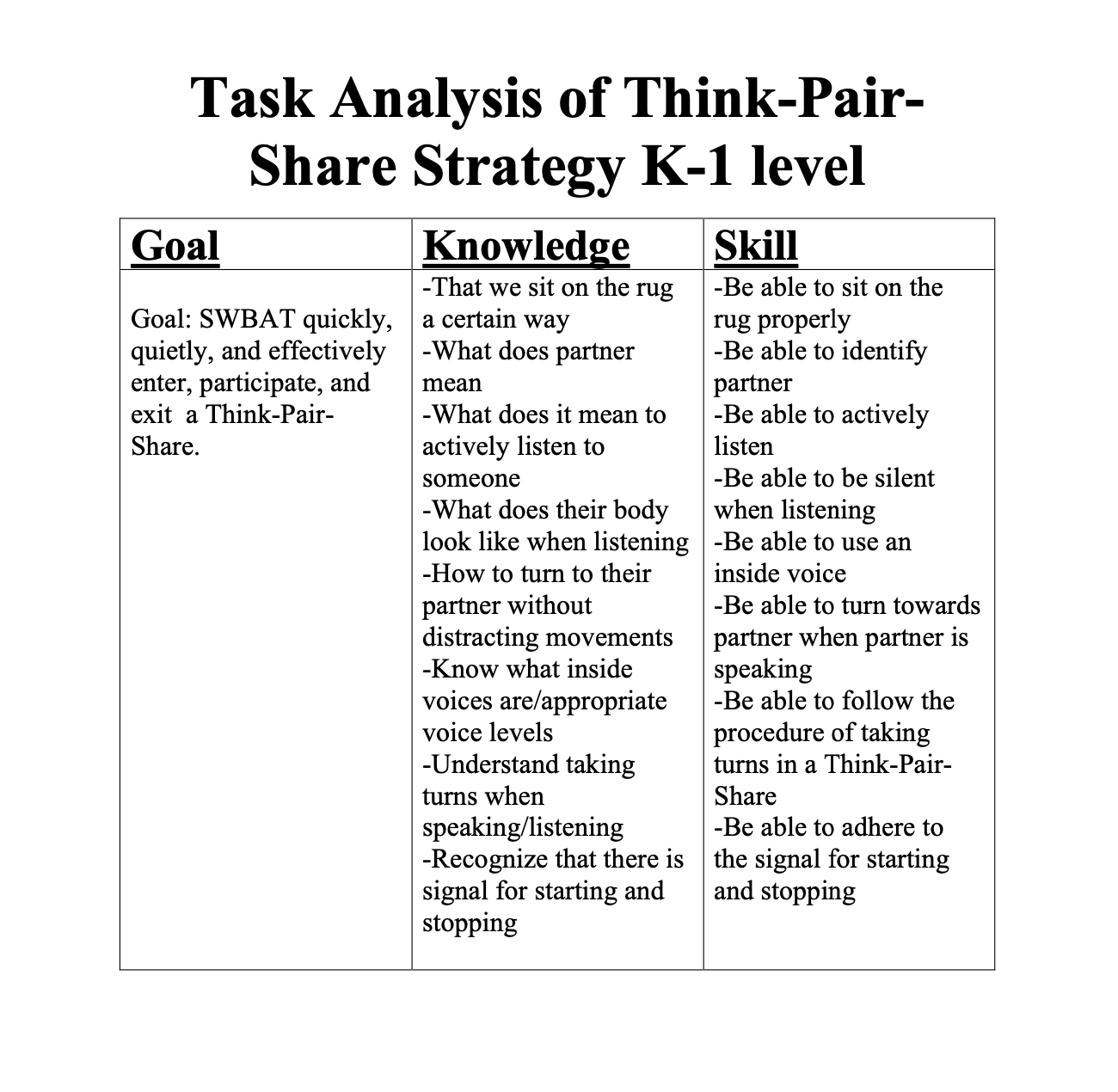
Purposeful Pacing for Community
While IM supports teachers by including lessons in every course that specifically target community building, IM trusts teachers to continue building this community throughout the school year. The curriculum provides regular opportunities for collaborative work and mathematical discourse throughout units, sections, lessons, and activities. Teachers must make the most of these opportunities to enhance their math communities. Creating and implementing routines, procedures, and learning goals surrounding grade-level speaking and listening standards can be a huge help.
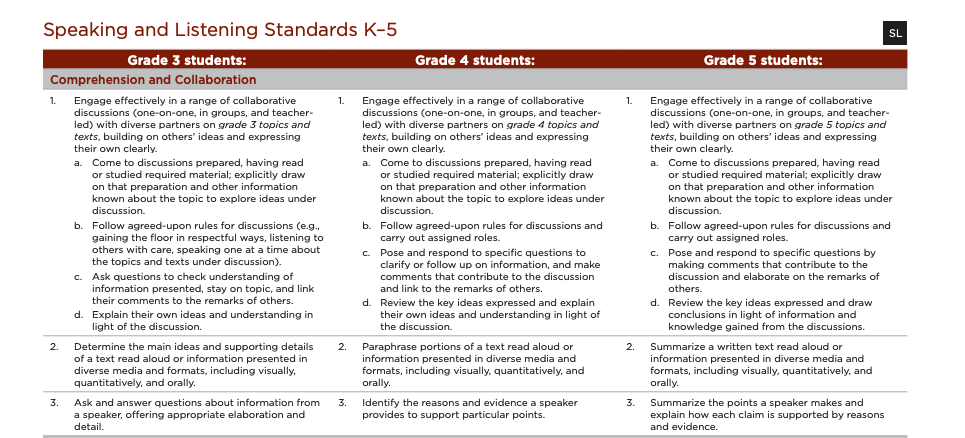
In addition to reflecting on Teacher Moves to Support Math Community, teachers can familiarize themselves with and highlight Common Core State Standards grade-level speaking and listening standards to support a strong math community. Creating a four- to six-week pacing plan that focuses on specific speaking and listening goals will allow teachers to target and explicitly teach essential skills that make up a math community. Just like any content area goals, teachers can follow the cycle of inquiry process to plan and collect, analyze, and use data to adjust instruction. Teachers can use a status of the class worksheet, like the one below, to track essential skills in their classroom math communities.
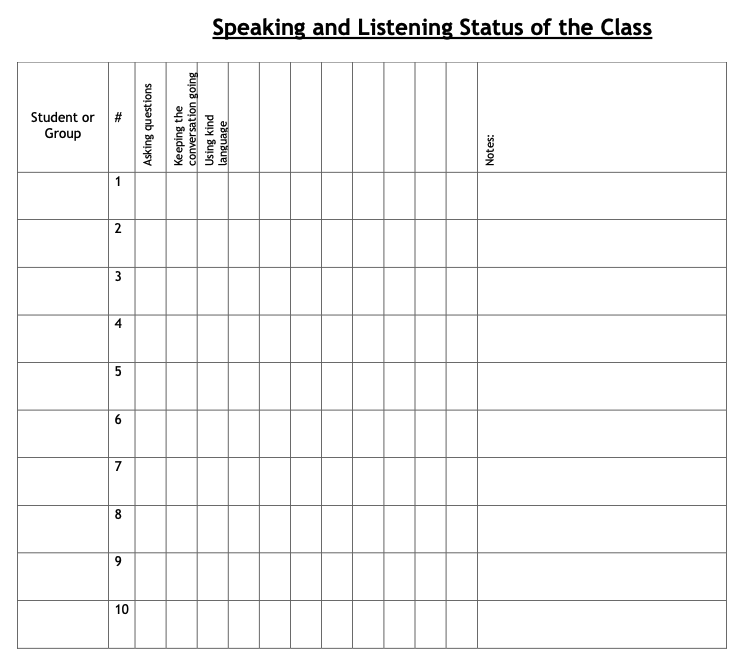
To start, the teacher identifies a specific community-building goal or focus for each week, plans how to teach and reinforce the goal, and determines how to assess students’ success in reaching the goal. Each learning goal should be paired with routines and procedures, such as think-pair-share, and student accessibility supports, such as discussion sentence starters or anchor charts. For example, the first week is a perfect time to work on listening skills, as it is discussed in all grade levels. Starting in first grade, Common Core State Standards use the terminology “listening with care.” Whether I was in the classroom or coaching teachers, I started the first week with explicitly teaching “listening with care.” In partnerships, students always had to respond with “I heard you say . . .” or other sentence starters. Students had to show self-control and only respond in a way that showed they were actively listening to what their partner was saying.
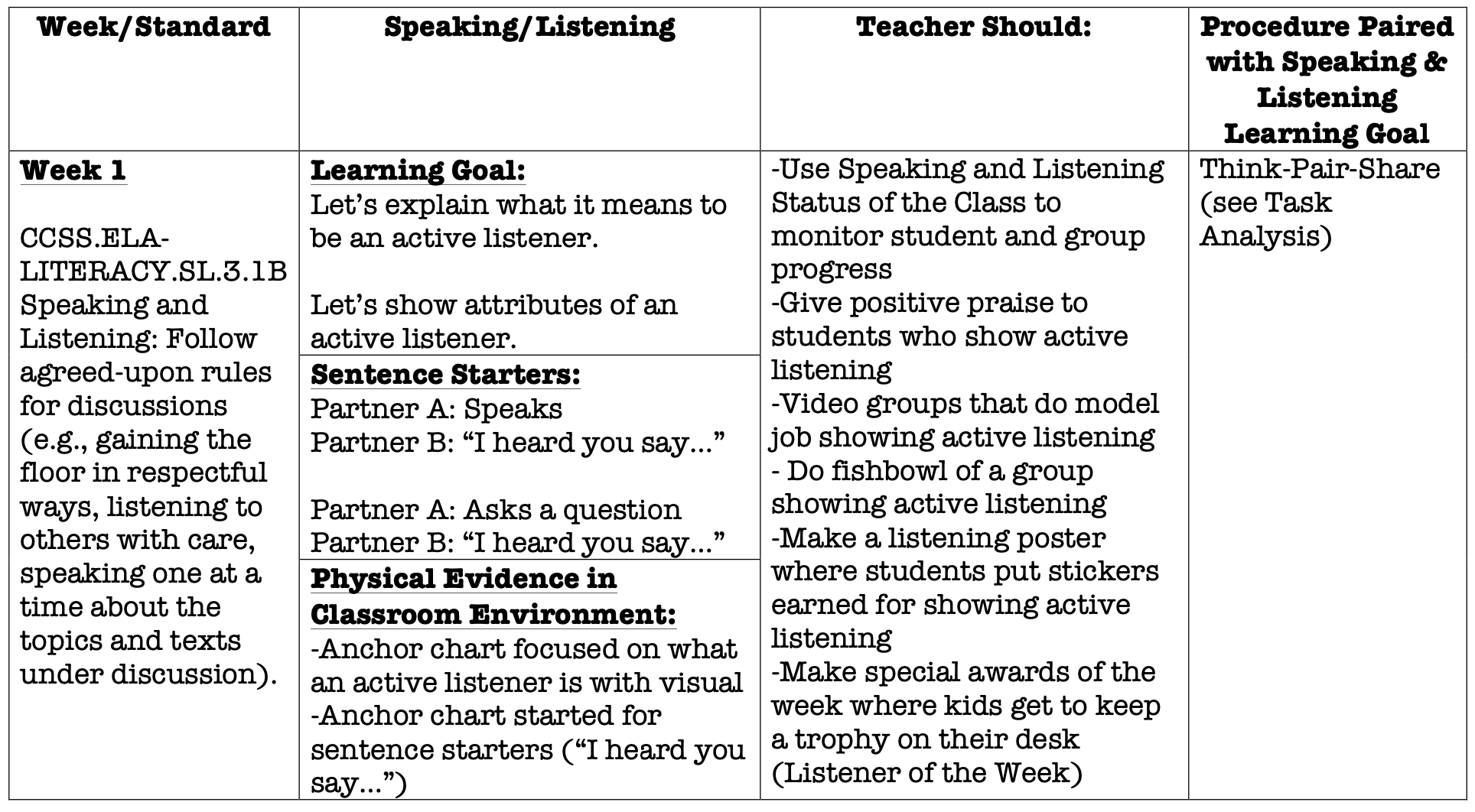
My last blog post, Leveraging PLCs to Maintain the Magic in Math Communities throughout the Entire Year, discussed how to use PLC times as opportunities to reflect on practices and plan for thriving classroom communities. Designating PLC time every four to six weeks for teachers to reflect on their math community data, discuss how they will adjust their instruction, and plan future math community instruction is an invaluable use of PLC time.
Conclusion
Planning for building a strong, authentic math community is like winter in Chicago. You cannot just plan for winter at the very beginning of the season. You have to plan for each snowfall separately. While planning at the beginning of the year to create an authentic math community is vital, this planning needs to be continuous throughout the year following a cycle of inquiry to adjust instruction based on the strengths and needs of a classroom’s math community.
Whether you are reading this blog at the beginning, middle, or end of your school year, the time is always right to start planning for or improving your math community. While the content of our lessons is important, let’s not forget the heart of Illustrative Mathematics lessons is the math community. As instructional leaders, let’s make sure we are providing teachers with time and space to prepare for greatness in their classrooms.
Author’s note: Special thanks to Pablo Gomez. The content of this blog post wouldn’t be possible without the hours of planning and discussion Pablo and I had about creating authentic math communities in our classrooms.
Next Steps
- How can teachers reflect on their current math communities in their classrooms to strengthen the ones in their classrooms next year?
- Is there a way to provide time and space for teachers to observe the math communities of students they will have next year?
- How can teachers collaborate and feel supported in planning for math community before school starts?
- How can you create space in PLC time to continue the work of planning for math community throughout the entire year?
 Meredith Dadigan Abel
Meredith Dadigan Abel
K-5 IM Certified® Facilitator
Meredith began her career as a member of the Urban Catholic Teacher Corps at Boston College, where she taught fifth grade while working toward her master’s degree. She then worked for 14 years as a teacher and dean for Aspire Public Schools in California. While at Aspire, Meredith received the California Charter School Teacher of the Year Award and became a semi-finalist for The New Teacher Project’s Fishman Prize for Superlative Classroom Practice. As dean, she coached teachers in improving authentic academic discourse in math classrooms. She now loves being an IMCF and helping educators implement IM in schools.
